
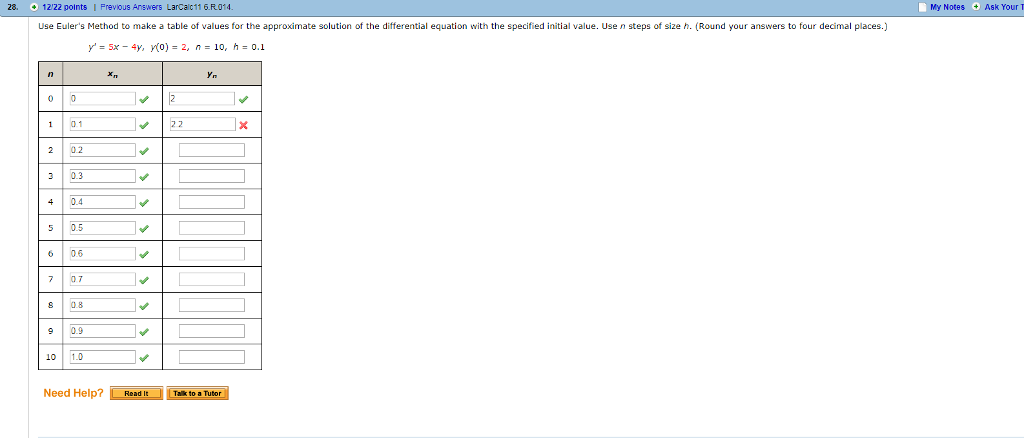
In the above examples, where n 7, there phi (n) 6, &. It represents the total count of numbers which are coprime to n. Khalid and Ali 3 introduced and investigated three new classes of integers by using the notion of Eulers totient function. NUMBER THEORY - Exercises - Warmups - 10 Compute \( \phi(999) \). For any number n, Euler Totient Function will be represented as phi (n). A graph of the number of solutions of Lemoines Conjecture for odd numbers up to 6000.

Outline of proof: let A, B, C be the sets of nonnegative integers, which are, respectively, coprime to and less than m, n, and mn then there is a bijection between A × B and C, by the Chinese remainder theorem. The counts of the number of solutions for 1,3,5,7. Si sint A et B numeri inter se primi et numerus partium ad A primarum sit = a, numerus vero partium ad B primarum sit = b, tum numerus partium ad productum AB primarum erit = ab. \( \varphi(mn)=\varphi(m)\varphi(n) \hspace gcd(m, n)=1 \) The totient function, also called Eulers totient function, is defined as the number of Positive Integers which are Relatively Prime to (i.e., do not contain any factor in common with), where 1 is counted as being Relatively Prime to all numbers. That is, if n is a, then ( n) is the number of integers k in the range 1 k n for which ( n, k) 1.
In number theory, Euler’s totient function, also be called Euler’s phi function \( \varphi(n) \) counts the positive integers up to a given integer n that are relatively prime to n. Graph of the first 100 valuesIn, Euler's totient or phi function, ( n) is an that counts the number of positive integers less than or equal to n that are to n.


 0 kommentar(er)
0 kommentar(er)
